Cutting an 11-tooth gear

M

mcdanlj

Registered
Registered
Joined
Aug 24, 2018
Messages
306
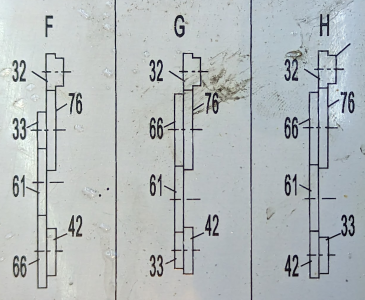
My first gear cutter set is on its way to me. I need an 11-tooth gear. I had a memory that 11 teeth was the fewest teeth you wanted on a standard 20° PA gear.

Now I see that the thinnest gear cutter (#1 or #8 depending on the convention used by the manufacturer) is specified for 12 teeth.

Oops.

My plan is to mesh with a 66-tooth gear for a 6:1 mechanical advantage. Can I reasonably quantify the impact of cutting an 11-tooth gear with the 12-tooth cutter?
 
Well, 66T/12T gives you 5.5 ratio with a "hunting tooth". I always criticize whole-ratio gears because they quickly establish wear patterns as the same teeth mesh over and over. Even on bikes, people would set up 3:1 and wonder why they kept wearing out chains. I ran a hunting tooth setup 45/17 and could go all season on a chain. That's why diff ratios are combination of primes: 4.11, 3.73, 3.55, so on.
 
Oh.

Wow I'm glad I asked for advice. TIL "hunting tooth"

I had been thinking of the 11-tooth gear as a wear item (in brass or aluminum against an existing steel gear) but even so, evening out the wear on the wear item is smart, and I doubt the different in advantage will matter to me. 12 teeth it is!

It won't hunt perfectly (common factor of 2) but it will at least wear more evenly. This is for an ELS particularly for thread cutting, so I'll trade off some wear for a non-repeating fraction, rather than look for a relatively prime ratio for a perfect hunting tooth ratio.

If I end up needing even more advantage, I guess I could cut a 120 tooth large gear and a 16 tooth small gear for 7.5:1 ratio that would also partially hunt.
 
Cutters are available for tooth counts of less than 12 teeth, they only work for one number of teeth less than 12. This is for range cutters, a hob can cut a correct gear for any usable number of teeth, but that is something most of us, including me, are not capable of doing.
 
pontiac428 said:
Well, 66T/12T gives you 5.5 ratio with a "hunting tooth". I always criticize whole-ratio gears because they quickly establish wear patterns as the same teeth mesh over and over. Even on bikes, people would set up 3:1 and wonder why they kept wearing out chains. I ran a hunting tooth setup 45/17 and could go all season on a chain. That's why diff ratios are combination of primes: 4.11, 3.73, 3.55, so on.
Click to expand...
Same thing with differentials in cars/trucks. Either the ring or the pinion is a prime number so the mesh pattern is essentially random.

Bruce
 
So that 66-tooth gear is one of the change gears for my G0709, and it normally meshes with a 33-tooth gear, so the normal arrangement doesn't hunt at all.

I may still do a 12-tooth gear, but also I do wonder whether I could double-cut with the #8 cutter to get enough undercut for an 11-tooth gear, if I work out the offsets. This feels like it shouldn't be hard if I spend some time with pencil and paper working out the geometry. Unfortunately, Machinery's Handbook (at least the 30th edition I have) seems to ignore metric gears altogether. ☹

Basically, I am thinking of something like this slitting saw gear cutting approach, but not needing lots of passes to approximate the involute, just adding undercut to avoid interference.

 
You could use a form cutter with the correct profile ground in?

Use one of the online gear generators, or plugin for CAD. Print your 11 tooth gear 1:1. Cut out, template on a piece of HSS and have at it grinding your form tool. Removing most of the material with something like a slitting saw before going in with the form tool isn't a bad idea.

Spur gears are readily available commercially, so that might be an easier option.
 
I've already printed and installed an 11-tooth gear here. It's possible that a printed gear could last a long time in actual use.

But it does seem that if I cut an 11-tooth gear with the 12-tooth #8 cutter, I should be able without modifying any of the full set of cutters I bought do additional cuts with the #8 cutter to add the needed undercut. Math should be higher resolution than a 3d printed prototype.

I think to keep the root rounded without stress risers, I would
  1. Cut all 11 tooth gaps as if I had an 11-tooth cutter
  2. Turn the gear backwards a bit (math) and move the gear cutter up a bit (math) and maybe away? (math) and cut 11 more times
  3. Turn the gear forwards twice as much (math) and move the gear cutter down similarly (math) and cut 11 more times.
The servo it is on isn't capable of much torque, so excessive undercut wouldn't actually be much of a problem.

Oooh, for working out the math, I'll bet I could generate 11-tooth and 12-tooth gears in the FreeCAD gear workbench, and then use sketcher constraints to make the computer do the math for me!
 
If I overlay the 11- and 12-tooth gears, I realize there is no need for additional undercut. The tooth is about 0.1mm thinner at the tip, and the involute is slightly moved.

1689373536789.png

So I can reasonably translate/rotate to put the tips together and line up the involutes at one side:

1689374027074.png


That's the quill down 0.15mm and turning the gear 1° clockwise.

So I think a working recipe would be:
  1. Cut 11 teeth normally using the dividing head. (on 40:1 dividing head: index circle 33; 3+21)
  2. Shift the quill down 0.15mm, turn the gear 1° clockwise, then make 11 cuts to cut away the extra bottom of the tooth above
  3. Shift the quill up 0.3mm, turn the gear 2° counterclockwise, then make 11 cuts to cut away the extra top of the tooth below
That should be near enough as makes no difference.
 
I've learned a few things:
  • I now understand why the idler gear in my change gear set has 61 teeth; it hunts perfectly with the other gears that it engages with.
  • There are gear pairs in my quick change gearbox that don't hunt much. In particular, the R and T settings select 1:2 or 1:2 ratio gear pairs, and the A setting has a 2:5 ratio.
  • I'm currently using a 3D printed 11-tooth gear (from before I asked this) as I'm testing, and it has way more mechanical advantage than I need, so I'm likely to change ratio and not care about cutting an 11-tooth gear after all anyway. I do think that the procedure I outlined here would work to cut an 11-tooth gear with a #8 cutter, though!
Here are the change gears. Every option hunts perfectly if I did the math right.
1689436188955.png

Characterizing the G0709 quick change gearbox shows all the gear pairings, some of which don't hunt perfectly, and a few of which hunt very little.
 
You must log in or register to reply here.
Back
Top