- Joined
- Feb 23, 2016
- Messages
- 480
I have 3D printed some gears in Fusion 360 using GF Gear Generator, with 50 and 25 teeth, Module 1.5mm, Pressure angle 14.5 deg, Helix angle 15 deg. The resulting large wheels are OD 78.5mm and small one is 40.6mm. How can I calculate spacing, meaning OC distance for them to mesh correctly? Is there a quick calculator? I have Machinery's Handbook open at "Gearing" and will slowly work my way through, but would happily take any advice to short cut the process.
By the way this is for an encoder attachment to a PM 728 mill that has a PDB.

Version 1 looks like this. There is a top lid, the bearings are on the bottom side, and the Omron encoder connects from the top intot the farthest wheel.
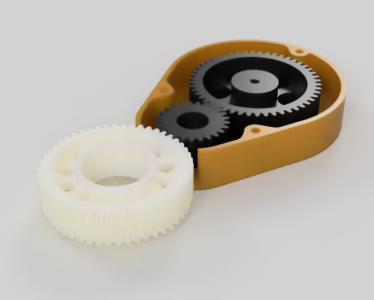
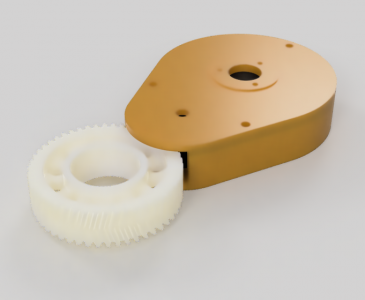
By the way this is for an encoder attachment to a PM 728 mill that has a PDB.

Version 1 looks like this. There is a top lid, the bearings are on the bottom side, and the Omron encoder connects from the top intot the farthest wheel.


Last edited:

